To find an antiderivative of
f(
y), we go through
our list of integration methods:
- Recognize elementary antiderivatives
- Rewrite the integrand to make it easier
- Use substitution to reverse the chain rule or simplify the
integrand
- Use integration by parts
- Use inspection to see the value of a definite
integral
to find one that works for this integrand. In this case,
only one method is appropriate. We use substitution to rewrite the integrand in terms of
w = cos(2
y).
This is shown below.
Substitution:
Let
w = cos(2
y). Then
w' = -2 sin(2
y), so
(-1/2)
dw = sin(2
y)
dy.
The integral can therefore be rewritten as
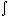
sin(2
y)
ecos(2y) dy =
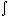 ew
ew (-1/2)
dw =
-(1/2)
ew +
C
Thus, substituting back for
w,
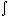
sin(2
y)
ecos(2y) dy =
-(1/2)
ecos(2y) +
C
To evaluate the definite integral, we take this antiderivative,
evaluate it at the endpoints of the integral (0 and pi/4),
and take the difference of the values. This gives
[ -(1/2) ecos(2(pi/4)) ] - [ -(1/2) ecos(2(0)) ] = (e - 1)/2.